Dear Aspirants, Our IBPS Guide team is providing new series of Quantitative Aptitude Questions for LIC AAO/SBI PO 2019 so the aspirants can practice it on a daily basis. These questions are framed by our skilled experts after understanding your needs thoroughly. Aspirants can practice these new series questions daily to familiarize with the exact exam pattern and make your preparation effective.
[WpProQuiz 5465]Click Here to Take LIC AAO Prelims Mock Test
Click Here for SBI PO Pre 2019 High-Quality Mocks Exactly on SBI Standard
Click Here to View Quantitative Aptitude Questions in Hindi
Directions (1 – 5): Each question below contains a statement followed by Quantity I and Quantity II. Find the relationship among them. Mark your answer accordingly.
a) Quantity I > Quantity II
b) Quantity I < Quantity II
c) Quantity I ≥ Quantity II
d) Quantity I ≤ Quantity II
e) Quantity I = Quantity II (or) relationship cannot be determined
1)
Quantity I: Simple interest earned on a certain sum of money after 4 years at the rate of 8 % per annum is Rs. 17920. Then find the principle amount
Quantity II: Rs.45000
2)
Quantity I: 2/5 minutes
Quantity II: A train can crosses a tree in 8 sec and the same train crosses the platform of length 150 m in 18 sec. Then find the time taken by the bridge of length 210 m?
3)
Quantity I: The area of rectangular garden is 2420 Sq m. The length of the rectangular garden is 25 % more than the breadth. Find the perimeter of the garden
Quantity II: The area of a square park is 2304 Sq m. Find the perimeter of the park
4) A bag contains, 5 pink, 6 yellow and 3 white balls.
Quantity I: If 3 balls are drawn randomly, then find the probability of getting at least one yellow ball?
Quantity II: 9/13
5)
Quantity I: Raji can swim at 8 km/hr in still water. The river flows at 3 km/hr and it takes 6 hours more upstream than downstream for the same distance. How far is the place?
Quantity II: 40 km
Directions (Q. 6 – 10): Study the following information carefully and answer the given questions:
The following table shows the percentage distribution of total number of students who completed their graduation from different universities and the ratio of male to female among them.

6) Find the total number of male graduates from the given universities together?
a) 29246
b) 25782
c) 33567
d) 37895
e) None of these
7) Total number of female graduates from university Q and R is approximately what percentage of total number of male graduates from university P and T?
a) 25 %
b) 40 %
c) 70 %
d) 50 %
e) 35 %
8) Find the ratio between the total number of male graduates from university Q, R and T together to that of female graduates from university P, R and S together?
a) 8576: 7115
b) 9254: 8471
c) 7280: 7777
d) 5890: 4783
e) None of these
9) Total number of graduates from university P and Q together is approximately what percentage more/less than the total number of graduates from university R and S together?
a) 5 % less
b) 5 % more
c) 15 % more
d) 15 % less
e) 30 % more
10) In which university has the highest number of female graduates?
a) University P
b) University Q
c) University R
d) University S
e) University T
Answers :
Direction (1-5) :
1) Answer: a)
Quantity I:
SI = PNR/100
17920 = (P*4*8)/100
P = (17920*100)/32 = Rs. 56000
Quantity II: Rs. 45000
Hence, Quantity I > Quantity II
2) Answer: a)
Quantity I:
2/5 minutes = 2/5 * 60 = 24 sec
Quantity II:
Let the length of train be x,
Speed is equal. So,
x/8 = (x + 150)/18
9x = 4x + 600
5x = 600
X = 120
The length of train = 120 m
Speed of train = 120/8 = 15 m/s
Required time = (120 + 210)/15 = 330/15 = 22 sec
Hence, Quantity I > Quantity II
3) Answer: a)
Quantity I:
The area of rectangular garden = 2080 Sq m
Length = (125/100)*breadth
l/b = 5/4 = > l : b = 5 : 4
5x*4x = 2420
20x2 = 2420
X2 = (2420/20) = 121
X = 11
Length = 55 m, Breadth = 44 m
Perimeter of the garden = 2*(l + b) = 2*(55 + 44) =2*99 = 198 m
Quantity II:
The area of a square park = 2304 Sq m
Area (a2) = 2304
Side (a) = 48
Perimeter of the park = 4a = 4*48 = 192 m
Hence, Quantity I > Quantity II
4) Answer: a)
Total number of balls = 5 + 6 + 3 = 14 balls
Quantity I:
n(S) = 14C3 = (14*13*12)/(1*2*3)
The probability of getting at least one yellow ball = 1 – P (None is yellow ball)
P (None is yellow ball)
n(E) = 8C3 = (8*7*6)/(1*2*3)
P(E) = n(E)/n(S) = 8C3 /14C3
= > [(8*7*6)/(1*2*3)] / [(14*13*12)/(1*2*3)]
= > 2/13
Required probability = 1 – (2/13) = 11/13
Quantity II: 9/13
Hence, Quantity I > Quantity II
5) Answer: a)
Quantity I:
x/(8 – 3) – x/(8 + 3) = 6
x/5 – x/11 = 6
6x/55 = 6
X= 55 km
Quantity II: 40 km
Hence, Quantity I > Quantity II
Directions (6 – 10):
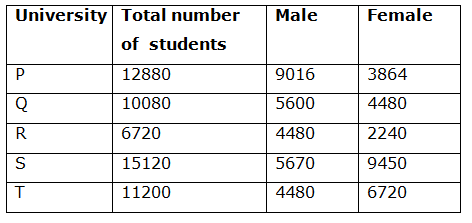
6) Answer: a)
The total number of male graduates from the given universities together
= > 9016 + 5600 + 4480 + 5670 + 4480
= > 29246
7) Answer: d)
Total number of female graduates from university Q and R
= > 4480 + 2240 = 6720
Total number of male graduates from university P and T
= > 9016 + 4480 = 13496
Required % = (6720/13496)*100 = 49.79 % = 50 %
8) Answer: c)
The total number of male graduates from university Q, R and T together
= > 5600 + 4480 + 4480 = 14560
The total number of female graduates from university P, R and S together
= > 3864 + 2240 + 9450 = 15554
Required ratio = 14560: 15554 = 7280: 7777
9) Answer: b)
Required % = {[(23 + 18) – (12 + 27)]/(12 + 27)}*100
= > {(41 – 39)/39}*100 = 5.128 % = 5 % more
10) Answer: d)
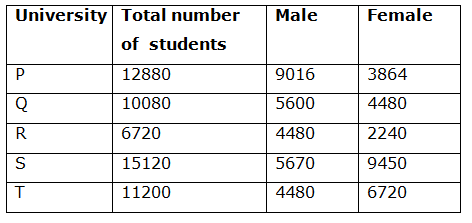
University S has highest number of female graduates.





